Flow past a solid sphere with different thiickness layers of air.

Upper half: Flow past a solid sphere resulting in a vortex. Lower half: Suppression of vortex due to an attached plastron.

Flow through a tube with a plastron with recirculating air.

Easing Flow: Plastron models

Why does the terminal velocity of a slowly rising bubble of gas in clean water not obey the same rules as the terminal velocity of a solid sphere falling slowly in clean water?

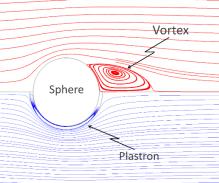
The flow of water past the bubble "rubs" its surface and sets the gas into a recirculating motion. The bubble has less drag because it can ease its way through the water. Hadamard-Rybzinski drag governs the drag of bubbles unless their surfaces are rigid (in which case Stoke's drag applies). The same effect can ease flow past solid spheres and across solid surfaces.

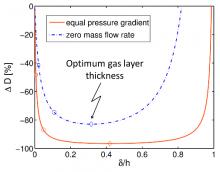
Our analytical models of the drag on an air encapsulated sphere at creeping flow predicts an 18% drag reduction in water. Numerical models predict that when the flow rate is increased the reduction in drag will be dramatic due to the suppression of vortices and the reduction in the wake. The same ideas can be applied to flow in channels and tubes. A recirculating layer of air between the solid and the liquid can lubricate the flow of the core liquid. Because a thin air layer cannot easily have an internal recirculation and a thick air layer obstructs the flow, there is always a best thickness of air layer.

Publications